
Content Partner
Grades Higher Education, 9-12

Don't have an account yet? Sign up for free
Don't have an account yet? Sign up for free
Nearpod version available
Students will be able to:

Students will explore graphs to analyze the impact of progressive, regressive, and proportional tax structures on families.
PREPARATION: students can take the quiz (Activity 1) the day before the lesson is taught or as warm up for the lesson
Taxation is the process in which a charge is imposed upon a taxpayer by government. Taxes are compulsory payments to governments by households and businesses. Tax structures impact households with different incomes differently. Representations of tax structures serve as a context for creating tables, graphs, and equations. An equation is a mathematical sentence that contains an equal sign. Equations use constants and variables to represent relationships between quantities. Variables are symbols used to represent numbers. We can analyze the impact of tax structure on households with different incomes by creating tables, graphs and equations. In the case of taxes, the average tax ratio can be represented as slopes of line segments between the origin and points of interest on graphs, representing relationships between income and tax.
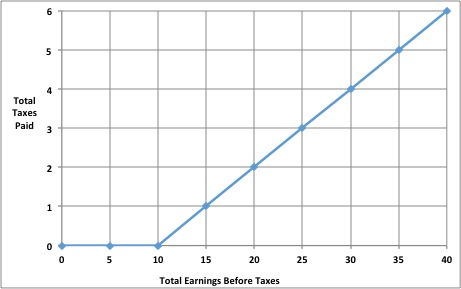
T = 0, when 0 ≤ I ≤ 10
T= .2(I-10)
Tell students that the equations represent a piecewise function, a special function used when one equation cannot represent all of the data provided.
[Students’ tables should be filled in as follows.]
|
Total Earnings Before Taxes |
Total Taxes Paid |
Average tax ratio |
|
$5 |
$0 |
0 |
|
$10 |
$0 |
0 |
|
$15 |
$1 |
0.066 |
|
$20 |
$2 |
0.10 |
|
$25 |
$3 |
0.12 |
|
$30 |
$4 |
0.133 |
|
$35 |
$5 |
0.143 |
REVIEW QUESTIONS
Multiple Choice
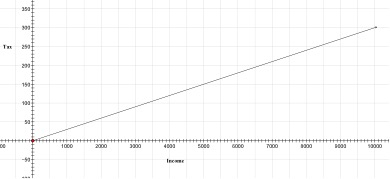
Constructed Response
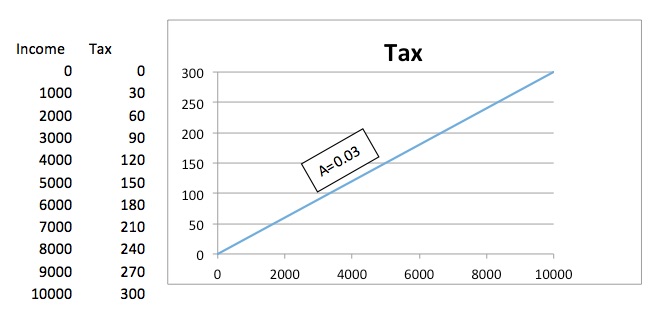
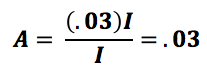
[Possible student answers: the proportional tax is fair because it taxes everyone at a constant ratio: the tax is fair because it takes a smaller amount from people with less income: the proportional tax is not fair because the amount paid by a lower income household leaves less disposable income than a higher income household: the tax is not fair because lower income households have to give up more necessities than higher income households.]

Content Partner
Grades Higher Education, 9-12

Grades 9-12

Grades 9-12

Grades 9-12
